Menu Close
  • Home
  • Blog
  • Differentiation Formula Tested in H2 A Level Math
    •

Differentiation Formula Tested in H2 A Level Math

Emily

Here are the differentiation formula tested in H2 A Level Math.

Properties of Differentiation

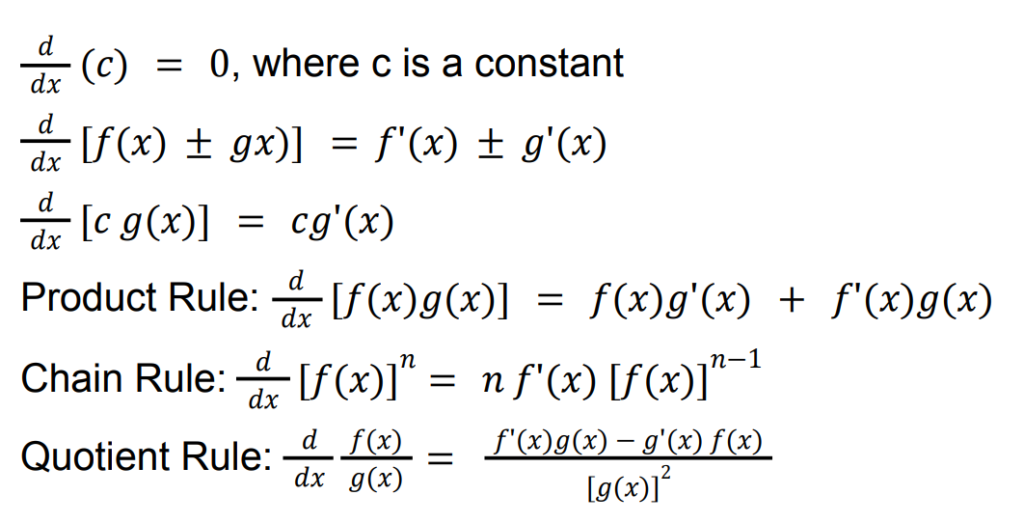
Here you'll find the formula for differentiation techniques , which includes differentiation of constant, product rule, quotient rule, chain rule, and so on.

Differentiation of algebraic functions

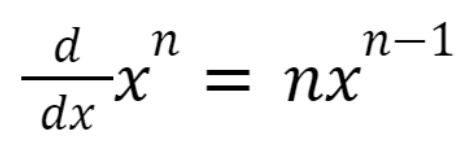
general formula for differentiation of algebraic functions

Differentiation of trigonometric functions

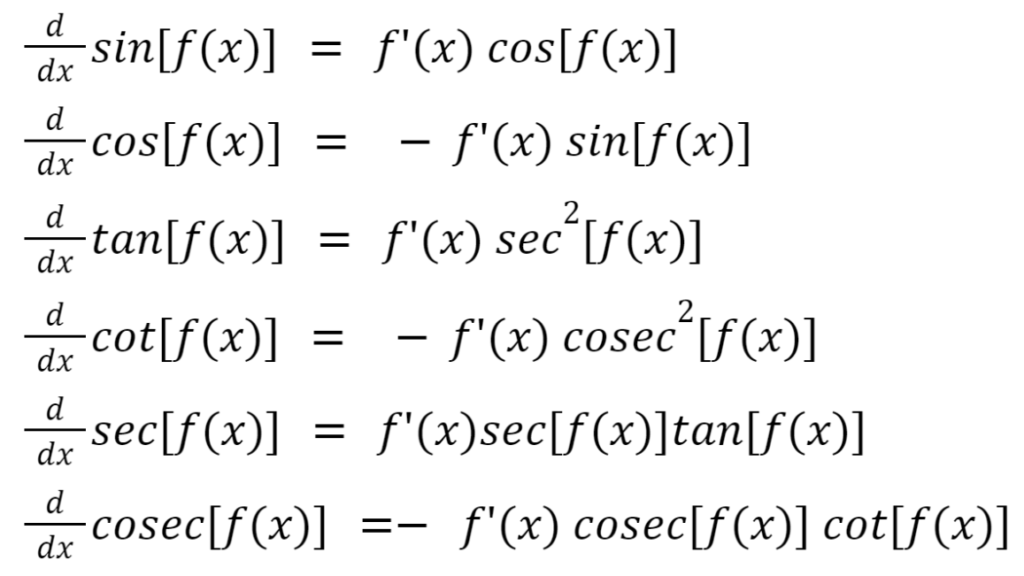
Here you'll find general formula for the differentiation of trigonometric functions, including sin x , cos x, cosec x, tan x, cosec x, sec x and more.

Differentiation of inverse trigonometric functions

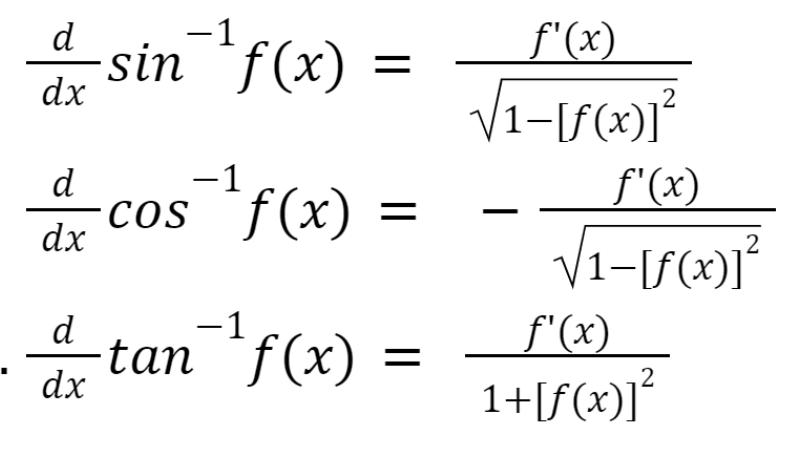
here are the formula for the differentiation of inverse trigonometric functions such as sin inverse, cosine inverse and tan inverse.

Differentiation of exponential functions

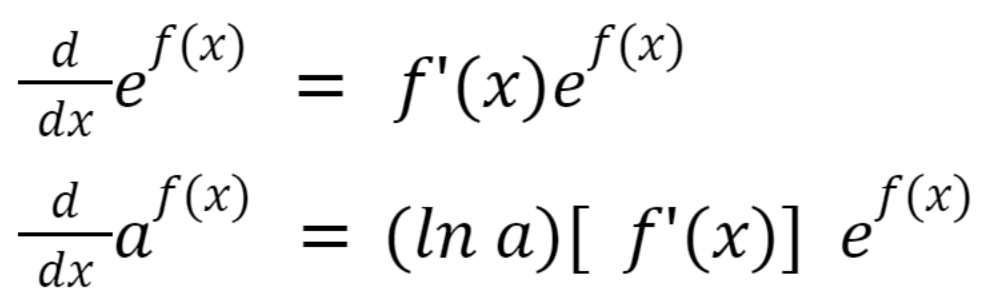
differentiation of exponential functions such as e to the power of x and a to the power of x.

Differentiation of logarithmic functions

differentiation of logarithmic functions such as ln x and log x.

Implicit Differentiation

Implicit differentiation is used when we do not have an equation expressed as y in terms of x. When that happens, we’ll use implicit differentiation to differentiate each term in that equation with respect to x.

Whenever we differentiate y with respect to x, we’ll get dy/dx.

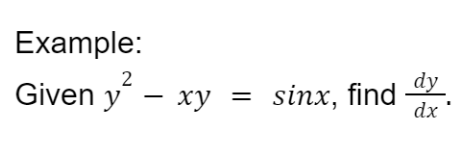
A question on applying implicit differentiation.
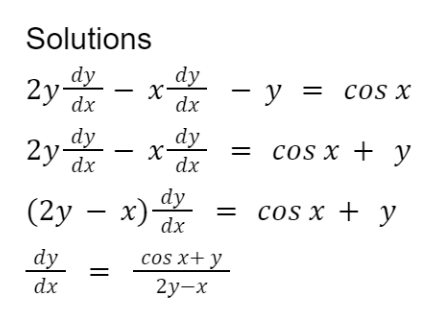
Solutions to a question on implicit differentiation.

Parametric Differentiation

Parametric differentiation is used when we have equations expressed in parametric form. I.e. x and y are expressed in 2 equations in terms of another parameter e.g. t.

When given a parametric equation, dy/dx can be found using chain rule.

how to find dy/dx of parametric equation. Here you'll find the formula to find dy/dx.
An example question with worked solutions on techniques of differentiation. This is a question on parametric equations, and finding dy/dx. In this question, we are given x and y in terms of t, and asked to find dy/dx.

Download a PDF Copy of Differentiation Techniques Summary

You can download a pdf copy of the differentiation techniques summary here.

Tagged
error: Content is protected !!